This page derives a geometrical and mathematical model for the lens and camera combination used most often in the Apollo lunar surface photography. This model then becomes useful in reconstructing the three-dimensional scenes captured in the photos.


VIEW VOLUMES

Aim your camera straight down at the floor. Look through the viewfinder and place an object on the floor at each corner of the area visible in your viewfinder. These objects represent the corners of a base of a pyramid. The apex of the pyramid is your camera lens. This pyramid is what can be called a "view volume". Everything your camera sees in this arrangement is inside that pyramid.

CLAVIUS

NASA: 71-H-1123 ANNOTATIONS BY CLAVIUS

When you aim your camera at real subjects, that pyramidal view volume is still there, but it's lying on its side. And instead of stopping at the floor, it continues on forever. The line coming straight out of the camera is what we will call the "optical axis". It's the line from the apex of the pyramid to the center of the base.

If we discover something about this view volume and learn to describe it mathematically, we can often use this information to help us understand what we see in photographs.

Your view volume is probably a little squashed. That's because video cameras and 35mm cameras use a rectangular frame format that's longer horizontally than it is tall. The Apollo Hasselblad cameras used a square frame which helps us out a lot.

The basic dimension of the view volume is the angle the pyramid walls make with each other. A narrow pyramid contains only objects near the optical axis while a wide pyramid includes all objects in the vague direction of photography. If we relate this to camera lenses, we find that telephoto lenses (and zoom lenses that are zoomed in tight) have narrow view volumes while wide-angle lenses have wide view volumes. This relates to the apparent magnification of the image. Both types of lenses record images on a frame of the same size. So if the contents of a narrow view volume are presented on a television screen, the individual objects appear larger in the frame. Similarly if the contents of a wide view volume are presented, each individual object appears smaller or farther away.

The manufacturers of camera lenses provide measurements that help the photographer determine the shape of the view volume. Usually the lens maker lists the "focal length" of the lens. If you know the size of the film frame, a formula coverts this to the "field of view" (FOV) angle. Sometimes lens makers will supply the FOV angle directly for certain film sizes.

The Zeiss Biogon lenses provided to NASA for the lunar surface cameras had a focal length of 60mm. This provides a FOV of 71° for the 70mm film frame supplied in the Kodak Ektachrome longroll.


A BIT OF TRIGONOMETRY

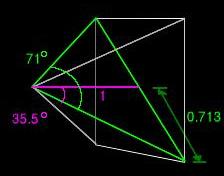
This 71° FOV angle is a diagonal FOV, measured from diagonally opposite corners of the frame. Going back to the pyramid example, draw your line from the lens to one corner of the viewfinder, then another line from the lens to the diagonally opposite corner of the viewfinder. Then measure the angle between these two lines. With the Zeiss Biogon lens this angle would be 71°.

This isn't very practical for measuring angles in photos. We're more interested in horizontal and vertical angles -- the angle between opposite faces of the pyramid. Fortunately a little trigonometry helps us.

CLAVIUS

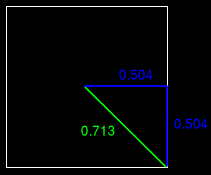
The view volume pyramid's diagonal angle is 71°. We arbitrarily set the height at 1, and we note that the angle from the pyramid's axis to any of the edges is 35.5° -- half the diagonal angle. It also creates a right triangle between the apex, the center of the base, and the corner of the base.

This allows us to compute the distance from the center of the postulated base to any of the base corners as the trigonometric tangent of 35.5° -- 0.713. This also forms the hypotenuse of an isosceles right triangle lying in the pyramid base.

CLAVIUS

The Pythagorean theorem allows us to compute the length of the sides of this right triangle, 0.504. The angle from the optical axis to the center of the edge is the arctangent of this length (since we arbitrarily defined the length of the axis as 1), or 26.75°. Therefore the horizontal (and vertical, since the frame is square) FOV is twice this angle, or 53.5°.

NASA: AS16-116-18716. ANNOTATIONS BY CLAVIUS

HAVING FAITH

The Apollo lunar surface cameras were fitted with reseau plates that overlays a grid of small crosshairs, called "fiducials", on every photo. The center fiducial represents the optical axis and it's a little larger than the others. The reseau grid can help us locate objects in the photo, and sometimes even to measure them. A moment's thought tells us that a fiducial corresponds to a certain angle from the optical axis. And if we can measure objects relative to the fiducials, we can determine their angular size, or the difference in angle between them.

We know from our work above that the horizontal and vertical FOV for the Biogon lens is 53.5°. But in most of the photos the edge of the image is not a reliable indicator of the full FOV. The film can jiggle around in the magazine, or slip in the camera. But we still need a way to relate the fiducial coordinate system with the theoretical edges of the frame.


AN EDUCATED GUESS

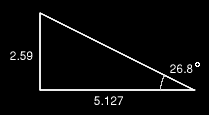
When confronted with a problem we can't solve analytically, we have to resort to empirical methods. That is, we have to measure some stuff and hope we measure enough so that any errors cancel each other out.

First we consider the reseau grid to be a coordinate system. The origin is the optical axis, the center fiducial. One fiducial to the right is 1.0 on the x-axis, while one fiducial down is -1.0 on the y-axis. It then follows that the edge of the photo should have some value in that coordinate system.

We turn to Michael Light's excellent book Full Moon. Light claims to have preserved the entire transparency frame in his prints. So we can measure where he thinks his idea of the frame edge lies. It turns out that the edges of all his photos are 2.59 fiducials away from the center.

Next we turn to the Apollo Lunar Surface Journal (ALSJ), which maintains online a repository of scanned Apollo transparencies. The JPEG images it makes available contain the entire frame, plus a surrounding black border. Picking 20 images at random and measuring where the image border falls in the fiducial coordinate system, we find that Light's 2.59 fiducials is consistent with ALSJ usage.

So if 2.59 fiducials corresponds to 26.8° (half the horizontal or vertical FOV), then we have another right triangle we can use to measure any angle in the photo whose distance is represented in the fiducial coordinate system.

The other leg of the triangle is computed as 5.127 (fiducials), meaning that for an object measure at F fiducials left, right, up, or down from the center fiducial, the corresponding angular difference, A, from the optical axis is given by the formula

A = arctan ( F / 5.127 )